Medium
1M
22
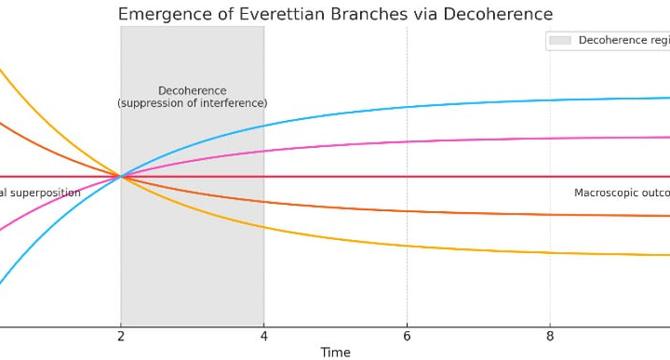
Image Credit: Medium
Decoherence and Everettian Branching: A Rigorous Treatment in the Caldeira — Leggett Model
- The combination of unitary Schrödinger evolution with a system — bath model leads to decoherence, einselection, and envariance, resulting in the Born rule for branch weights in the Many-Worlds Interpretation.
- A derivation using the Caldeira — Leggett model shows the exponential suppression of off‐diagonal terms, the emergence of pointer states defining Everettian branches, and the satisfaction of the Born rule for branch weights.
- Decoherence in a quantum particle coupled to harmonic oscillators exhibits rapid suppression of off-diagonal elements, leading to the vanishing of coherence between spatially distinct states.
- Pointer-state selection via einselection emphasizes that only states diagonalizing the interaction Hamiltonian remain robust, leading to the formation of localized position basis for classicality.
Read Full Article
1 Like
For uninterrupted reading, download the app